In the SU(3) chiral soliton (Skyrme) model, there are predictions
of a variety of light (A=2,3,4)
clusters with multiple strangeness, which could be stable with respect
to strong and possibly even weak non-leptonic decays [46, 47].
This form of the soliton
model is consistent with the masses of strange and non-strange
baryons (A=1). These predictions are very speculative, but
sufficiently dramatic to merit an experimental test. These objects
are distinct from strangelets, in that binding is already possible
for very small A and negatively charged states are as stable as
positively charged ones. They are also distinct from six quark bag
states (e.g., the H) since soliton states of isospin 
are
bound (ex. 
, with I=2). Some of the possible
dibaryon (A=2) soliton states are indicated in Table 
,
together with the energy release Q in weak decay processes for
systems bound at the strong decay threshold.
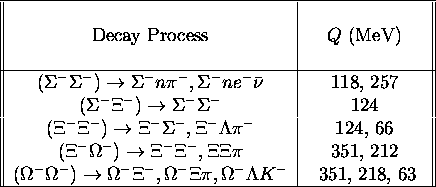
Table: Multiply strange chiral solitons and the predicted energy
release, Q, for their weak decays (assuming zero binding).
The binding energies of the above A=2, S=-2 to -6 states are
quite model dependent, but could be as much as 20% of the rest mass
in the Skyrme model. As seen from Table , a binding energy of 5%
is already sufficient to stabilize the A=2, I=2, J=0 bound state
against mesonic weak decay. Such an object could
be detected by E864: it is negatively charged with 
and it requires only two units of
strangeness for its production, a more favorable situation than for
strangelets. Note that these A=2 systems will not be strongly
bound by conventional long 
and medium 
range meson
exchange. Their existence would represent a dramatic confirmation of
the short range chiral dynamics of the SU(3) soliton picture. The
dynamics of the six quark bag, with one-gluon exchange treated
perturbatively, produces a different level order for the A=2
system. In the bag, the color magnetic energy is minimized for SU(3)
flavor representations of minimum dimension, and hence I=2 states
like are unbound. The search for negatively
charged stable dibaryons is as fundamental as that for the neutral
H. It bears directly on the dynamics of baryon-baryon interactions
at short distances, a region where the meson exchange picture breaks
down, and a correct treatment of quark degrees of freedom becomes
crucial. E864 can provide significant limits: if the
state exists, it should be produced with easily
measurable cross section in Au-Au collisions at the AGS. The other
objects in Table require more strangeness production, with
correspondingly smaller cross sections.
Systems with A=3,4,5 and several units of strangeness are also
predicted to be bound [47]. Attractive
cases for E864 include 
and
. Again, one can show that these objects are
not bound by long range forces (single pion exchange plus second order
pion tensor interactions), so their existence would be a dramatic
indication of significant attraction in the short-range interaction.
The weak decays of these objects have not been estimated. For
weak decay, Donoghue et al. find a
lifetime significantly longer than that of the 
, because the
enhanced 
weak interaction, responsible for the 
rule, does not enter for a s-wave decay (the 
,
transition dominates) [44].
Similar effects may occur for
some of the other A=2 systems. For instance, the 
bound state is a member of the 
-plet of SU(3), while
states occur for and 
. Thus in some
cases, the weak lifetimes could be sufficiently long ( 
ns or
so) for the particles to be detected by E864, even if the state is
bound by less than the Q value shown in Table .